Numerical relativity simulations of compact binaries: comparison of cell- and vertex-centered adaptive meshes
Given the compact binary evolution problem of numerical relativity, in the finite-difference, block-based, adaptive mesh refinement context, choices must be made on how evolved fields are to be discretized.
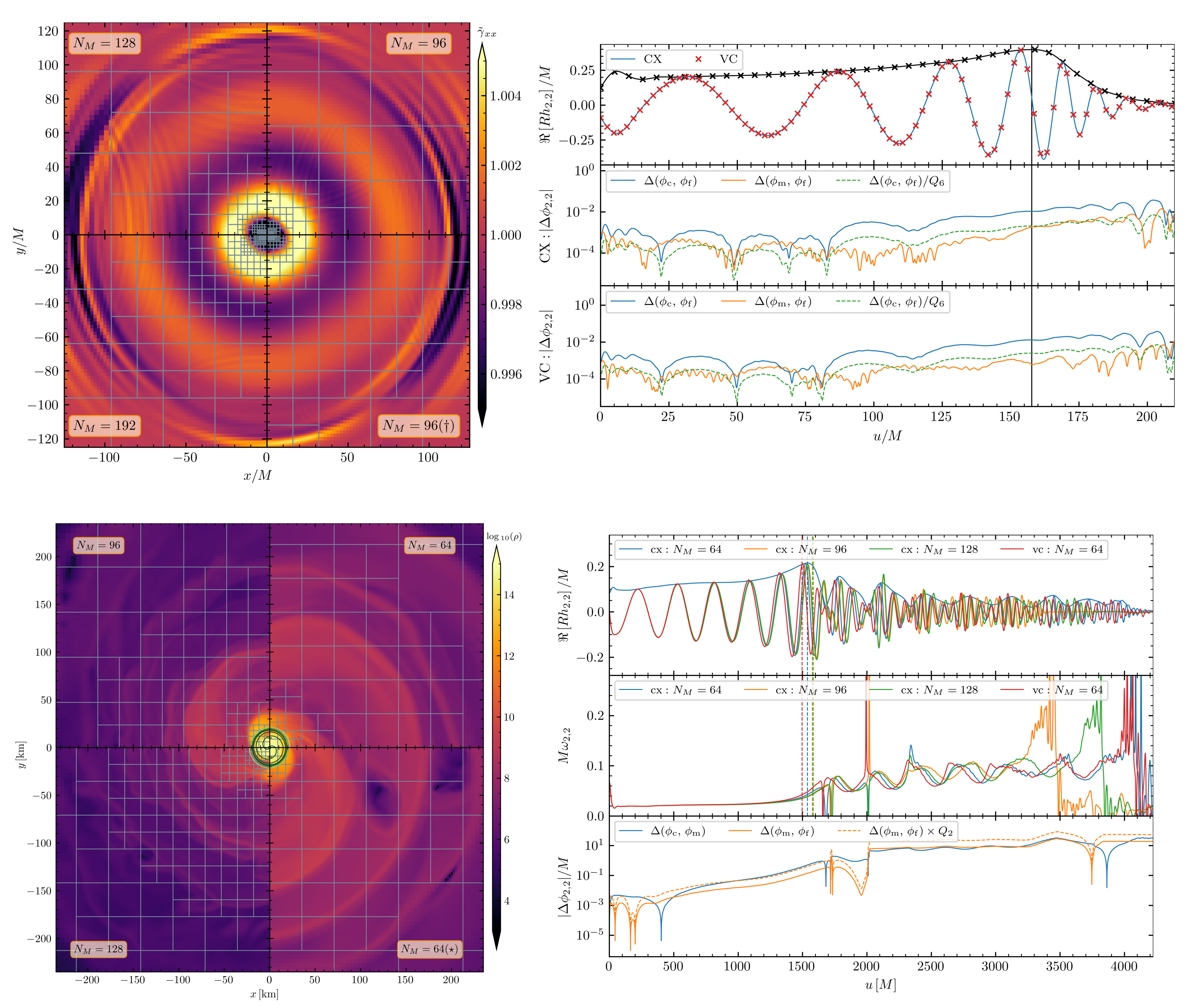
In GR-Athena++, the space-time solver was previously fixed to be vertex-centered. In 2406.09139 our recent extensions to a cell-centered treatment, are described. Simplifications in the handling of variables during the treatment of general relativistic magneto-hydrodynamical (GRMHD) evolution are found. A novelty is that performance comparison for the two choices of grid sampling is made within a single code-base. In the case of a binary black hole inspiral-merger problem, by evolving geometric fields on vertex-centers, an average ~20% speed increase is observed, when compared against cell-centered sampling. The opposite occurs in the GRMHD setting. A binary neutron star inspiral-merger-collapse problem, representative of typical production simulations is considered. We find that cell-centered sampling for the space-time solver improves performance, by a similar factor.
Read more